 To Foot
To FootRemember to include, where necessary, the effects of the rotation of the primary.
Take a small satellite mass m, planet mass M, density D, planet radius R, orbit radius R (no atmosphere...), period t, angular velocity ω, gravitational constant G.
Balancing "centrifugal" and "gravitational" forces,
m ω2 R = G m M / R2
→ ω2 = G M / R3 ;
Mass = volume×density : M = 4/3 π R3 D
→ ω2 = 4 π G D / 3 ;
Period : T = 2 π / ω →
T = √ ( 3 π / (G D) ) seconds.
The field outside a homogeneous sphere depends only on its mass and the distance; not on the size of the sphere.
So one can now see that the period of an unpowered satellite in circular orbit depends ONLY and inversely on the square root of the average density within its orbital sphere; this assumes spherical symmetry, but not otherwise homogeneity. Interesting?
The result relates immediately to Kepler's Third Law, that T2 is proportional to R3.
Now I have an OLD book to hand, so forgive the cgs units :-
G
= 6.673E-8 cm3 gm-1 sec-2 and
D = 5.53 gm cm-3
∴ G D
≈ (20/3 E-8) × (11/2) = (110/3) E-8 sec-2
∴ T2 ≈ 3 π / (G D)
≈ 3 (22/7) / ((110/3) E-8) sec2
≈ 25×106 sec2
∴ T ≈
5×103 sec = 5000 sec ≈ 84 minutes ...... OK!
More accurately, T ≈ 5054 sec = 84 min 14 sec.
Approximately, the radius of the earth is 4000 miles,
and the atmosphere is 100 miles deep;
so the average density within a final orbital
height is D × (4000/4100)3 ,
so the final period is about
T × (4100/4000)1.5
= T × (1 + 1/40)1.5 ≈ 87 min 24 sec.
The Moon's density is 3.39 gm/cc; low lunar satellites take about two hours per orbit (if ground-hugging : 107.5 minutes).
A spherical non-cohesive body would break up if it were rotating in less time than that given by the expression above.
So, assuming, for example, nearly lunar density: don't plan on driving all the way around an asteroid in less than about two hours.
The stability of such an orbit, in an inverse-linear field, is another question entirely.
Starting with Section 2.4 of A S Ramsey's "Newtonian Attraction" (CUP)†, and using only a little additional mathematics, it appears that the corresponding expression for a low orbit of an infinite circular rod is similar : T = √ ( 2 π / (G D) ) seconds
o
/|
/θ|
/ |
/ |
=====≡=======
† Uses the angle θ of the element from the particle when integrating along the rod, making integration simple. The total attraction of a finite rod seems proportional to the sine of half the angle that it subtends at the particle, divided by the perpendicular distance.
The diagram shows part of a horizontal infinite circular rod of density ρ, an inscribed sphere and its circumscribing cylinder, with their centre line and an orbiting particle virtually touching the rod. The curved surfaces S of the sphere and of the cylinder have the same area. The volume V of the cylinder is 3/2 times that of the sphere.
Consider the surface fields F at the particle, from the whole rod and from the sphere alone. By symmetry, the surface fields of the sphere and of the rod are uniform and perpendicular to the surfaces, and the field of the rod across the plane surfaces of the cylinder is zero.
Now div F = k ρ and ∫ F dS = ∫ div F dV. So the surface field of the cylinder is 3/2 times that of the sphere, and the low orbit period for the rod is therefore √(2/3) times that of the sphere.
The period of ANY circular orbit is inversely proportional to the square root of the average density within the minimum circumscribing sphere (assuming spherical symmetry).
For low lunar orbit, time is around 108 minutes, density is, say, 3.39; so it looks like 0.138 days (12 kiloseconds) divided by the root mean density.
It applies also to planets, of course; but as the average density is so low (for Earth's orbit around Sol, about (0.7×106/150×106)3 of the volume is of mean density 1.4 and the rest is virtually empty) the figures get harder to handle.
Consider two satellites in orbits differing only slightly in radius; the inner will overtake the outer.
Natural logs → 2 ln T = ln k + 3 ln R
differentiate → 2 dT / T = 3 dR / R
Also, dS / S = dT / T
→ dS / S = 3/2 × dR / R
For one orbit, S = 2 π R
→ dS = 3 π dR ~ 10 dRWhat is the tangential separation change per revolution?
Let R be the orbital radius, T be the period, and S be the distance along the circular orbit. For a given primary, k will be a constant. Let dR be the difference in height of the two orbits and dS be the tangential separation change.
Ten times as much, nearly; the result holds for all circular orbits in inverse-square fields.
See also in Gravity 2.
If an object in a circular orbit about a point primary were stopped, it would follow half of a degenerate elliptical orbit of half the major axis. The time to fall to the centre would therefore be 0.5×(0.5)1.5 ~ 0.177 times the time to orbit (cf. "Jupiter Five", A C Clarke; "Hector Servadac", Ch.X, Jules Verne) :-
One can see that the duration of a Hohmann transfer between circular orbits must be more than 0.5 times the period of the inner body, and within the range 0.177..0.5 times the period of the outer body. The maximum wait for starting such a transfer seems to be the reciprocal of the difference of the reciprocals of the periods.
See also in Gravity 2.
The simplest Beanstalk is a (very) high strength massless cable from Earth's surface to a large mass in (circular) Geosynchronous Earth Orbit. Clearly, if one falls off from near the bottom of the cable one will hit the Earth, and if one falls from near the top one will remain in a permanent elliptical orbit. GEO is about R=26300 miles from the centre of the Earth; the top of the atmosphere is at about K=4100 miles. From what height, X, does a fall give an atmosphere-grazing orbit?
Let GEO speed be U, release (apogee) speed be V, grazing (perigee) speed be W; let the mass of the Earth be M and let the gravitational constant be G. At apogee and perigee, the velocity is horizontal. Conservation of energy in orbit means K.E. plus P.E. is constant.
We need, and get, extreme solutions X=K=0 and X=K=R; and infinite K gives X3 = 2R3, X = 21/3R, plausibly (Note : that's only Earth escape).
Equation (c) can readily be solved numerically by the box below (which uses the method of Find Zero), giving 18700 miles from the centre of the Earth.
Confirmed by Richard Kennaway, 2001-05-29, news:rec.arts.sf.science. See also Arthur C Clarke, The Fountains of Paradise, Chapter 19.
Distances must be in consistent units, and may be given as expressions. A solution is sought within the X range given; the minimum must exceed K and the maximum must be finite.
The above algebra shows that, dropping out from a radius of 21/3 ≈ 1.260 times GSO on a beanstalk, one just manages to fall to infinity (ignoring solar gravity, which matters).
If, however, one slides out along a frictionless beanstalk from GSO, one must reach local escape velocity before reaching that radius.
Integrate the difference between centrifugal and gravitational forces out from GSO, combine the velocity with the tangential. TL, in news, got escape velocity by sliding out to a radius of (3/2)1/2 ≈ 1.225 times GSO.
The general character of the path of a moon, as seen from above its orbital plane, is determined only by the relative masses of its sun and its planet, and the relative orbital radii of the planet and the moon.
For determining the character in a specific case, one can use the periods instead of the masses; the calculation then applies also to non-orbiting objects, such as points on planetary surfaces.
The following calculations and figures are approximate and need checking.
Take the orbits as being co-planar*, and take the orbit of a secondary to be a circle centred on its primary. Let the distance of a moon from its planet be r, that of the planet from its sun be R; let the mass of the planet be m, and the mass of the sun be M; let the orbital speeds and periods of moon & planet around their primaries be v & V and t & T.
* : Think carefully about the Uranian system ...
The path of a moon which orbits in the same direction as its planet will cross itself if, when the planet is between its moon and the sun, the orbital speed of the moon more than nullifies that of the planet. Retrograde moons act conversely. For a given planet, moons within a certain radius X will have self-crossing paths.
The speed of a moon is given by v2/r = Gm/r2; thus v2 = Gm/r. Equating v2 to a similar V2 for the planet gives X = R×(m/M).
The speed of a body is ωr and ω = 2π/T. Therefore, X = R×ω/Ω is the maximum distance from the planet for a moon's orbit to be self-crossing.
Orbits :- Moon : radius 400,000 period 1/12 so speed ~ 12×400,000 Earth : radius 150,000,000 period 1 so speed ~ 150,000,000
The Earth's orbital speed around the Sun is many times that of the Moon round the Earth. so the Moon's true path is not self-crossing.
Points on the surface of a planet will self-cross if its Day is less than rY/R, where r is now its radius and Y its Year. Saturn just qualifies; Jupiter nearly does.
The path of a moon will be everywhere concave towards its sun if its orbit is so large that, when the moon is between the planet and the sun, the pull of the sun exceeds that of the planet. For a given planet, moons outside a radius C will have all-concave paths. This is rare; but our Moon's path is so.
The field of the planet at the moon is Gm/r2; that of the sun at the moon is GM/(R-r)2 ~ GM/R2; equating these fields, C = R×√(m/M) is the minimum distance from the planet for a moon's path never to be convex towards its sun.
The acceleration of a circling body is ω2r and ω = 2π/T. Therefore, C = R×(ω/Ω)2 is the minimum distance from the planet for a moon's orbit never to be convex towards its sun.
Orbits :- Moon : period 1/12 radius 400,000 so field ~ 150×400,000 Earth : period 1 radius 150,000,000 so field ~ 1×150,000,000
The field at the Moon from the Earth is about 40% of that from the Sun, so the Moon's true path always curves towards the Sun.
Only long-known moons are considered here. Except for Jupiter, only the outer satellite need be considered for possible all-concavity. Except for Jupiter and Saturn, only the inner satellite need be considered for possible self-crossing.
Taking the Sun to have mass 2×1030 kg, and putting ---- where X is less than the planetary radius, and putting "+" for satellites with all-concavity and "-" for self-crossers :-
Planet Xmax Cmin Satellite
R MMi m kg MMi MMi Extremes r MMi
Earth 93 6.0E24
---- Moon 0.238
0.161 Moon 0.238 +
Mars 142 0.6E24
---- Phobos 0.0058
0.078 Deimos 0.0146
Jupiter 483 1.9E27
0.46 Europa 0.417 -
14.9 Hades 14.7
Saturn 886 5.7E26
0.25 Dione 0.235 -
15.0 Phoebe 8.05
Uranus 1763 8.8E25
0.08 Miranda 0.076
11.6 Oberon 0.364
Neptune 2793 1.0E26
0.14 Triton 0.22
19.7 Nereid 3.50
Pluto 3666 1.3E22
---- Charon 0.012
0.296 Charon 0.012
NOTE that these data are from old books - masses from WHJ Childs (1958), distances from P Moore (1958) (except Pluto/Charon).
Earth: the Moon is easily far enough out for total concavity.
Jupiter: Hades is nearly far enough out for total concavity.
Jupiter: Amalthea, Io, Europa ; Saturn Rings, Mimas, Enceladus, Tethys, Dione are self-crossers.
The path of the Moon around the Sun is everywhere concave towards the Sun; the Solar pull on the Moon always exceeds that of the Earth, by a modest factor. It has much the same shape as a very well-worn threepenny bit; one with no flats left on its sides, but still not circular.
The fields are proportional to m/r2;
in kg / mile2 :-
. Sun ≈ 2×1030 /
(93×106)2
≈ 2.3×1014
. Earth ≈ 6×1024 /
(0.24×106)2
≈ 1.04×1014
Or, by centrifugal force, they are proportional to
rω2.
For r in miles and ω in units of 2π/yr :-
. Sun ≈ 93×106 × 12 =
93×106
. Earth ≈ 0.24×106 × 132
≈ 41×106
These ratios are in reasonable agreement.
(N.B. lunar mass neglected.)
The mass of the Moon is about 1/81 times that of the Earth; hence the barycentre, about which both orbit, is 240,000/82 = nearly 3,000 miles from the centre of the Earth, about 3/4 of the way to the surface.
In its annual path around the Sun, the Moon travels only slightly further than the Earth does.
Integration is over a time given in both Months and Years, which should each be integer. There should be many steps per month. Defaults suit Moon and Earth; 254 sidereal months in 19 years would be more exact.
The path of Charon is NOT everywhere concave towards the Sun; the Solar pull on Charon is seven hundred times less than that of Pluto.
The fields are proportional to m/r2;
in kg/km2 :-
. Sun ≈ 2×1030 /
(6×109)2
= 5.5×1010
. Pluto ≈ 1.3×1022 /
(2×104)2
= 3.3×1013
Or, by centrifugal force, proportional to
rω2; in km/(yr)2 :-
. Sun ≈ 6×109 / 2502 =
9.6×104
. Pluto ≈ 2×104 × (365/6.4)2
= 6.5×107
These ratios are in reasonable agreement.
(N.B. Charon mass and Pluto orbital eccentricity neglected.)
The mass of Charon is about 1/7 times that of Pluto; hence the barycentre, about which both orbit, is 20,000/8 = nearly 2500 km from the centre of Pluto, over two radii.
Pluto's orbital velocity is 4.74 km/s, while that of Charon is 0.23 km/s; hence Charon's orbit around the Sun is not locally self-crossing.
The Galactic Year is of the order of 250 My, and Sol is of the order of 30 kly from the Core; we are about 1/7 light-hour from Sol. (Revise figs? : 200 My, 25 kly ?)
Without a figure for the effective mass of the Galaxy, one cannot directly get the gravitational field; but one can compare the components of the net acceleration of the Earth - ω2R, proportional to R/T2 where T is the period.
In light years per year-squared :-
E-G : 30,000 / 250,000,0002 = 3×104 /
6×1016 = 5E-13
E-S : 1/(365×24×7) / 12 = 1.6E-5
so, if those figures are anything like right,
E-S exceeds E-G by a factor of the order of 3×107
--- which is actually less than I'd expected, but safely > 1.0.
The Earth's path is not everywhere concave to the Galactic centre.
The velocities are proportional to R/T :-
S-G : 30,000 / 250,000,000 = 3×104 /
2.5×108 = 1.2E-4
E-S : 1/(365×24×7) / 1 = 1.6E-5
so, if those figures are near enough right,
S-G exceeds E-S by a factor of the order of 8, and the Earth's
path is not self-crossing.
The binding energy, U, of a sphere (homogeneous, of mass M and radius R) is the energy required to move all of its particles to infinity in different directions; it is given by U = 3/5GM2/R.
To calculate U, one can dismantle the sphere shell by shell and integrate; from the above, the energy dU required to move a shell of thickness dr and mass dm to infinity from a surface at radius r and local gravity g is given by dU = g r dm.
We have g = GM(r/R)3/r2 and dm = M×3r2dr/R3 (volume increases thrice as fast as radius, relatively). Thus :-
U = ∫0R
[ GM(r/R)3/r2
× r × 3Mr2dr/R3 ]
U = 3 G M 2 / R6 ×
∫0R
[ r4dr ] = 3/5 GM2/R
Alternatively, the gravitational potential energy of the sphere (zero after disruption) can be calculated.
The potential energy dU of such a shell around a mass M' = M(r/R)3 is dU = G M' dm / r. Thus :-
U = ∫0R
[ G × M(r/R)3
× 3Mr2dr/R3 / r]
U = 3 G M 2 / R6
× ∫0R
[ r4dr ] = 3/5 GM2/R
The energy required to move one particle m from the surface at R to infinity is E = mgR, and there g = GM/R2, so E = GmM/R ; the binding energy is the energy required to lift all the material, in constant surface gravity, to a height of 60% of the radius.
The central pressure of a self-gravitating fluid sphere of radius R and uniform density ρ is
P = 2/3 π G ρ2 R2
The expression is dimensionally correct. The G and ρ2 terms are reasonably obvious; and the field within a sphere is proportional to the distance from the centre, and the amount of material within a parallel-sided column is proportional to its length, suggesting R2.
For that and other material, see Physics topics : Celestial Mechanics by Dr. J. B. Tatum of the University of Victoria, Canada.
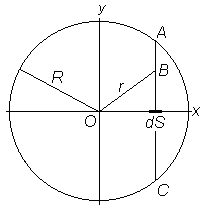
The figure shows such a sphere in cross-section.
Consider a small element of area dS on the X axis and lying perpendicular to the Y axis, and the volume bounded by perpendiculars to its rim in the +y direction; the end of this volume is at A. The force on dS will be the sum of the components, in the -y direction, of the forces on the elements dy, which is the integral of (the field at y times the cosine factor times a mass of the element).
Inside a spherical shell, its field is zero; outside, its field is as if all its mass were at the centre. The field at B is Gm/r2 = G ( 4/3 π ρ r3 ) / r2 = 4/3 π ρ G r from the shells smaller than r.
P dS =
∫0Y (4/3 π ρ G r)
(y/r) (ρ dS dy)
= 4/3 π ρ2 G
[y2/2]0Y dS
= 2/3 π ρ2 G
Y2 dS
For the central pressure, where x=0, put Y=R to get P = 2/3 π ρ2 G R2
Alternatively, one can put dS in perpendicular to the X axis at r and integrate outwards, which gives the same result
P dS =
∫rR (4/3 π ρ G x) (ρ dS dx)
= 4/3 π ρ2 G
[x2/2]rR dS
= 2/3 π ρ2 G
(R2 - r2) dS
For the central pressure, where r=0 :- P = 2/3 π ρ2 G R2
These need checking.
Integrate the pressure over the plane dividing two hemispheres :-
F =
∫02π ∫0R
2/3 π ρ2 G
(R2 - r2) r dr dθ
F =
4/3 π2 ρ2 G ∫0R
(R2 - r2) r dr
F =
4/3 π2 ρ2 G
[R2r2/2 - r4/4 ]0R
F =
π2 ρ2 G R4 / 3
Integrate the pressure over the slice indicated by AC, at a distance x from O. Put Y = √(R2-x2), its radius.
F =
∫02π ∫0Y
2/3 π ρ2 G
(R2 - (x2 + y2)) y dy dθ
F =
4/3 π2 ρ2 G ∫0Y
(Y2 - y2) y dy
F =
4/3 π2 ρ2 G
[Y2y2/2 - y4/4]0Y
F =
π2 ρ2 G Y4 / 3
That at least agrees with the previous. Note that there is no dependence on the radius of the sphere.