 To Foot
To FootNewton was born early on Christmas Day 1642 (about 90 days before the end of the year 1642). That was Gregorian 1643-01-04, a Sunday. See Date Miscellany I.
From Hawking's cited book :-
To see whether those are Newton's actual words, see in the Principia.
In Newtonian gravity, every particle in the universe attracts every other particle with a force proportional to the product of their masses divided by the square of the distance between them.
Wikipedia, Gravitational constant, August 2009 :-
In SI units, the 2006 CODATA-recommended value of the gravitational constant is:
G = (6.67428 ± 0.00067) ×10-11 m3 kg-1 s-2
Those units are equivalent to N m2 kg-2.
The gravitational field due to a body of mass M at a location of distance r is the net gravitational force on a unit mass at that location, g = G M / r2 and the gravitational potential there is P = - G M / r with zero potential being at infinity.
For "particle", read "element of mass", and recall that energy and mass are related by E = mc2 so that fields also gravitate :- g = G E / (rc)2 where E is the total energy of the attracting point.
Then there's Special and General Relativity, and Gravity Waves, and Quantum Gravity : I disregard these things here, except to note that the current belief is that gravitational mass and inertial mass are always identical, whatever the nature of the mass may be.
I read that geosynchronous orbit radius is 42,164 kilometers. This implies a value for GM of similar accuracy; the individual values of G and M are less certain.
The average radius of the Earth is 6378 km.
A uniform spherical shell generates no internal field; the internal field of a homogeneous sphere is proportional only to its density and the distance from the centre. The external fields are as for a point mass.
Note that the arguments do not apply to the field within a ring or disc.
It has been said that : "the field inside a uniform spherical shell is zero (which is indeed so for any 1/r2 type force); a non-obvious, non-trivial result needing calculus for proof."
This is not so; the cancellation of field is correct, but there is no need for calculus. This is relatively well known in the case of gravitation, but not for electrostatics. In the following, "charge" stands for "electric charge", "mass", or any other source of an inverse square field; and "small" means "infinitesimal".
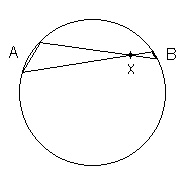
Consider an arbitrary point X inside a uniformly-charged spherical surface, and consider both an arbitrary small element of "charged" surface area A and that second small element of area B which is marked out by straight lines from the edge of the first area through the point to meet the sphere again on the opposite side of the point.
The solid angles subtended by A and B at the point X are equal, and the directions XA and XB are opposite. Simple geometry makes it clear that the areas A and B (which have the same "tilt") and hence the charges are proportional to the squares of their distances from the point X. Their fields therefore cancel at that point; so there will be no net field from the whole set of such areas which covers the whole sphere, for any point X.
The argument is correct; I think I have expressed it correctly. Ramsey (s.3.2) says that Newton used it, in Principia Proposition LXX; indeed, it is in Hawking's cited book, p.880.
The above would apply in electrostatics for the field inside a uniformly charged spherical shell. The argument for the field being zero inside a general conducting surface is different.
The field outside a spherical shell is as if the mass of the shell were concentrated at its centre.
The direction of the field is evident from symmetry; the magnitude at a large distance must be as for a point mass, and there is unlikely to be any other solution compatible with these.
An argument closely resembling that given for an internal point proves that the field at an external point due to the part of the shell visible from the point equals that due to the hidden part.

Ramsey (s.3.2) presents, by a simplified argument, Newton's result in Principia Proposition LXXI; Hawking p.881.
Let the shell have centre O, radius r and surface density m; its total mass M is 4πmr2. For any external point X, there is an internal inverse point Y, on the same radius and with OX.OY = r2. Let the length of OX be d.
At any point Z on the shell, let there be a small element of shell subtending solid angle dω at Y, with area ds = YZ2 dω / cos(YZO) and mass m ds.
OZ = r, OX/OZ = OZ/OY, and angles XOZ = ZOY; so triangles XOZ & ZOY are similar.
The radial field contribution at X from ds is diminished by cos(ZXO), and angles ZXO = YZO. The contribution is therefore G m (YZ/ZX)2 dω = Gmr2 dω / OX2 which, summed over the whole shell, becomes 4πGmr2 / OX2 = GM/d2.
The internal surface field is zero.
The external surface field of a shell of given areal density m is independent of the radius of the shell, 4πGm.
The field acting on the surface itself is half that; the method above applies, but the summation is over half of the solid angle.
A Lamina resembles the front part of an infinite spherical shell, which implies that its field is everywhere 2πGm.
A sphere may be considered as a nest of spherical shells; cf. Newton, Proposition LXXIV.
The field due to a spherically symmetrical body at any internal point is given only by the sphere of material nearer to the centre, since shells outside that distance have no net effect. If the sphere is also homogeneous, the shells inside that distance provide a total mass which is proportional to the density and the cube of the distance.
By the inverse-square law, the field at any point within a homogeneous sphere will thus be proportional to the density and to the distance from the centre.
The surface gravity g at the surface of a sufficiently symmetrical sphere is proportional to the product of the density ρ and the radius r :-
At a distance d from the centre the force on a mass m is mgr2/d2. Integrating this from r to infinity gives the escape energy as mgr2/r = mgr. Therefore, surface escape velocity is what would be needed to attain a height of one radius against the surface field.
Falling one radius in the surface field gives a velocity satisfying V2 = 2gr.
Consider two homogeneous spheres X & O of masses M1 and M2, separated by a distance r not much larger than the sum of their radii. Each is in an inhomogeneous gravitational field due to the other.
Is the force between them still G M1 M2 / r2 as for large r or for point masses? It needs proving.
The following argument, more or less, was earlier given by Newton for Propositions LXXV, LXXVI (Hawking pp. 884, 885).
It has been shown above in Field Outside a Spherical Shell that the external field of sphere X is as if its mass were concentrated at its centre. The force on sphere O is due to that field.
It looks as if one can consider sphere O as a nested set of shells, and then use an argument like that above to show that the force on each shell (equal and opposite to its force on X) is as if its mass were concentrated at the centre of sphere O; the axial force on the element Z is equal and opposite to that of Z on X.
The total force is thus G M1 M2 / r2 .
It is easily seen that the X and Y components of force on a secondary orbiting within its primary are directed to and proportional to the distance from the Y and X axes respectively. So both components of motion are simple harmonic with the same constant period independent of size. The motion is therefore elliptical, and the centre of the ellipse is at the centre of the primary. The outer limiting case matches low circular orbit, giving the period. The period-density relation is clearly consistent with this.
In particular : the period of an orbit within a homogeneous spherical primary is constant, depending only on the density.
For a homogeneous spherical airless primary, the times for a low circular orbit and for one full period of motion along any straight chord are the same. For Earth, the low-orbit time is close to 84 minutes.
Consider a homogeneous spherical non-rotating airless Earth, with necessary frictionless tunnels of negligible cross-section. Consider a ground-level satellite orbiting along the Greenwich Meridian, and a test probe of equal mass dropped into a pole-to-pole tunnel as the satellite passes. Consider the N-S axial components of the forces on each; these are obviously the same at the instant of release.
That on the satellite, resulting as it does from a constant radial force resolved, is proportional to the distance of the satellite from the equatorial plane.
That on the probe, being inverse-square dependent on the mass within its current distance from the centre, with that mass being proportional to the cube of the radius, is proportional to the distance from the centre.
These forces being the same, the N-S components of velocity and position for the two objects, initially matching, will continue to match.
Now consider, instead of the satellite, a second probe simultaneously dropped into a chordal tunnel; and remember that the angle in a semicircle is a right angle. Resolve now the forces on the two probes along the direction of the tunnel. Again they match, so the chordal components of the motions also continue to match.
In frictionless chordal motion within a homogeneous sphere, the transverse (perceived) force is constant, and proportional to the minimum distance from the centre.
To fall to the centre of a homogeneous Earth, from anywhere not outside it, takes about 21 minutes; for any other body, similar but inversely proportional to the square root of the density.
What are the greatest and least times required to fall to the centre of a spherically-summetrical but non-homogeneous body of a given mean density? It seems likely that they correspond to the cases where all of the mass is in the centre, and where the body is a spherical shell.
But how about the brachistochrone, the minimum-time path? There are certainly paths faster than the chord. The minimum time must increase from 0 to 42 minutes as the angular distance travelled increases from 0° to 180°.
It is perhaps easier now to consider the half-period, the time taken to traverse a chord, or other path, from surface to surface. Consider now the straight route from a non-equatorial point on the Greenwich meridian to the point at the same latitude on the opposite meridian, and compare with a straight-straight route under it with a bounce under the pole but above the centre (diagram may follow). Each half of this route is less than, and therefore quicker than, the corresponding part half of a full chord in the same direction; it is a faster route - but doubtless even the best (which I suspect is the one whose sections, produced, meet the equator) of these is not the optimum.
The optimum seems likely to be not far from an arc or a semicircle; but in the limit of a short path on a vast sphere must tend to a cycloid, it seems - the brachistochrone in a uniform field is a cycloid.
Scaling to a sphere of unit radius with mass such that LEO has unit angular velocity, at any point of the trajectory the velocity is given by the cosine of the distance from the centre. For the fastest path, the trajectory will be that of a light ray in a sphere of refractive index given by the secant of the distance, whatever that is.
An attempt to determine the function via a Lagrange's Undetermined Multiplier became too complex ...
2001-06-06 : I read that this is treated by John Prussing in Am. J. Phys., 44, #3, March 1976, pp. 304-305.