To Foot

The Geometry of Ellipses.

This page is ancillary mainly to Gravity Page 2, Kepler, where it originated.

Definitions of the Ellipse

By Conic Section

By a dictionary definition, an ellipse is the figure produced when a cone is cut by a plane making smaller angle with the base than the side of the cone makes (COD 1970).

The cone can in fact be a cylinder, and so an ellipse is a stretched circle, from which its Cartesian form follows.

By Directrix

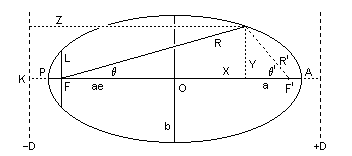
An ellipse is defined in plane geometry (Chambers: directrix, eccentricity) as the set of points (X, Y) such that the distance R from a given point F, termed the focus, is e times the distance of the point from a fixed line, the Directrix.

By Polar Equation

R = L / (1 - e cos θ)

By Cartesian Equation

(X/a)² + (Y/b)² = 1

By String Loop

An ellipse could also be defined as the path of a point whose distances R & R' from two fixed foci F & F' have a constant sum.

Consequent Geometry

This section is being worked upon, in the hope that it will be useful in discussing Kepler.

General Diagram

The order in which the parts of the diagram should be generated depends upon the definition chosen.

Conic Section

Directrix

An ellipse is the locus of points for which   R = eZ   where R is the distance from a given point F, e is a given constant, and Z is the distance from a given line, the directrix.

Let the distance between directrix and F be f ; then   f + R cos θ = Z = R/e , and so   R = ef / (1 - e cos θ) , the polar equation with L = ef .

Put the X axis through F and perpendicular to the directrix; put the origin O on it at a distance greater by ae where a is an unknown constant. Let the directrix be at X = -D ; then Z = D+X .

Setting   Y = 0   gives
  Apoapsis : R // +X :  X_a + ae = R = e(D + X_a)
  Periapsis : R // -X :  -X_p - ae = R = e(D + X_p)
Those are satisfied by the choices   X_a = -X_p = a = eD   which match the diagram above.

Setting   X = 0   in the definition now gives   R = a   then Pythagoras gives   b = Y = a(1 - e²)^½ then applying him to a general point   (X-ae)² + Y² = R²   which leads to   (X/a)² + (Y/b)² = 1   with   b² = a²(1 - e²) , and that shows the ellipse to be a stretched circle.

Since that has no odd terms in X, by symmetry there must be another directrix at   X = +D   and a point F' at   X = +ae   such that   R' = e(D-X) . Thus   R + R' = e(D+X) + e(D-X) = 2eD =2a , so an ellipse can be drawn with two pins, a loop of thread, and a pencil.

Cartesian

An ellipse is the locus of points for which   (X/a)² + (Y/b)² = 1   where a is the semi-major axis and b is the semi-minor axis. Its eccentricity is defined as   e = (1 - (b/a)²)^½   ;   b/a = (1 - e²)^½

The distance R from a point on the ellipse to the point (-aE, 0) satisfies   R² = (X+aE)² + Y² ; replace Y using the ellipse equation and then replace b using a and e and expand the products. If E = e and   D=a/e   the result simplifies to   e(X+D)  which is the directrix equation.

Focus-to-centre FO : ae
Semi-latus rectum L : Y at X = -ae : L = b(1-e²)^½ : L = a(1-e²)
Apoapsis FA : q = a (1 + e)
Periapsis FP : p = a (1 - e)
  so : p/q = (1-e)/(1+e)   ;   q+p = 2a   ;   e = (q-p) / 2a = (q-p) / (q+p)

Polar

An ellipse is the locus of points for which   R = L / (1 - e cos θ) ;  L, given by   θ = ± π/2, is the semi-latus rectum. Mirror-symmetry across the line   θ = 0   is obvious, and R cos θ = (R-L)/e .

Now   cos θ = (Z-K)/R   for some K, so
  R = L / (1 - e (Z-K)/R)   →   R - e (Z-K) = L   →   R = e (Z-K-L/e) ;
thus the perpendicular at   K = L / e   from F is a directrix.

The extremes of R occur when   cos θ = ±1 ; thus
Major axis : 2a = R_max + R_min = L / (1-e) + L / (1+e) = 2L / (1-e²) ; symmetry across this axis is obvious from the symmetry of cos θ .
Minor axis : differentiating, the maximum of R sin θ is at   cos θ = e : then   2b = 2L / (1-e²)^½ ; symmetry across this axis is not obvious.

A Theorem?

Consider two equal curves of unknown shape given by R = L / (1 - e cos θ) and R' = L / (1 - e cos θ'), with the angles being measured at F from a line FF' and at F' from the reverse line F'F. They are symmetrical about θ=0 and will be mirror images of each other in the perpendicular bisector of FF'. For a point common to both curves, FF' will equal the sum of the projections of the radii onto the line.
  FF'   =   R cos θ + R' cos θ'   =   (R-L)/e + (R'-L)/e = (R+R'-2L)/e
That must prove something.

String Loop

Take a loop of string of total length 2a(1+e) and two pins a distance 2ae apart; R+R' is constant.

Focusing

Since R+R' is constant, wave optics implies focusing of rays from one focus onto the other.

Since R+R' is constant, the tangent to the ellipse at that point makes the same angle with each of them; then geometrical optics implies focusing.

These are alternative views of the same situation.